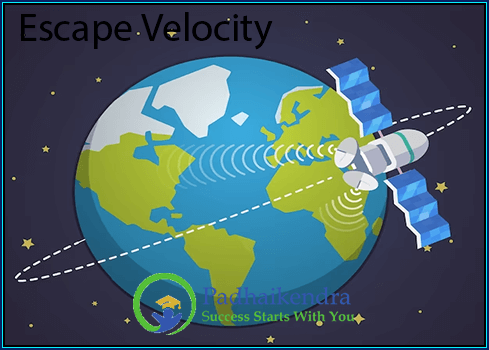
Escape velocity is the minimum speed that an object must attain in order to escape the gravitational pull of a planet, moon, or other celestial body. It is the speed at which the object’s kinetic energy is equal to its gravitational potential energy, allowing it to break free from the gravitational field and continue moving away indefinitely.
The escape velocity of an object depends on the mass and radius of the celestial body from which it is trying to escape. The formula to calculate the escape velocity is:
v = sqrt(2GM/r)
where v is the escape velocity, G is the gravitational constant, M is the mass of the celestial body, and r is its radius.
Example- The escape velocity of the Earth is about 11.2 kilometers per second (or 40,320 kilometers per hour).
This means that an object launched from the surface of the Earth must attain a speed of at least 11.2 km/s to escape the Earth’s gravitational pull.
Escape velocity is an important concept in space travel, as it determines the amount of energy and speed required to launch a spacecraft or satellite from a planet’s surface and send it into space. It is also used in the study of celestial mechanics to understand the behavior of planets, moons, and other celestial bodies.
Escape velocity FAQs
Escape velocity is the minimum velocity required for an object to overcome the gravitational pull of a celestial body (such as Earth) and completely escape its gravitational field.
Escape velocity can be calculated using the formula:
Escape velocity = √(2GM/r)
Where G is the gravitational constant, M is the mass of the celestial body, and r is the distance from the center of the body to the object.
No, escape velocity varies depending on the mass and size of the celestial body. For example, the escape velocity on Earth is different from the escape velocity on the Moon or other planets.
If an object achieves escape velocity, it will continue to move away from the celestial body it originated from and into space, with its trajectory determined by its initial velocity and other external influences.
In theory, any object can achieve escape velocity if it attains the necessary speed. However, it would require a significant amount of energy and propulsion to achieve escape velocity for massive objects like spacecraft.
The factors that affect escape velocity include the mass and size of the celestial body, the gravitational constant, and the distance from the center of the body to the object.
Escape velocity is the minimum speed needed to escape the gravitational field of a celestial body, while orbital velocity is the speed needed for an object to maintain a stable orbit around a celestial body.
Escape velocity is crucial for space exploration as it determines the energy and velocity required for spacecraft to leave Earth's atmosphere and reach other celestial bodies.
Yes, escape velocity represents the minimum speed required to escape a celestial body's gravitational field. Objects can achieve greater velocities, but they will still be influenced by the gravitational pull of other celestial bodies.
Yes, natural phenomena such as volcanic eruptions and meteorite impacts can propel objects to velocities that exceed escape velocity momentarily, but they are still subject to gravitational forces and eventually return to the celestial body.