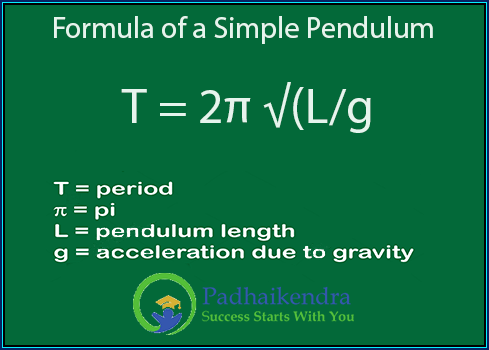
The formula for the time period (T) of a simple pendulum is:
T = 2π √(L/g)
Where:
T represents the time period of the pendulum, measured in seconds (s).
π (pi) is a mathematical constant approximately equal to 3.14159.
L represents the length of the pendulum, measured in meters (m).
g represents the acceleration due to gravity, approximately equal to 9.8 meters per second squared (m/s²) on Earth.
This formula states that the time period of a simple pendulum is determined by the length of the pendulum (L) and the acceleration due to gravity (g). It indicates that the time period is directly proportional to the square root of the pendulum’s length and inversely proportional to the square root of the acceleration due to gravity.
By using this formula, one can calculate the time period of a simple pendulum given its length and acceleration due to gravity. This formula is derived from the principles of harmonic motion and is applicable for small amplitude oscillations where the angle of displacement is relatively small.
This formula assumes that the amplitude of the pendulum’s swing is small so that the motion is approximately simple harmonic. It also assumes that there is no air resistance or other external factors that would affect the pendulum’s motion.
Note that the time period of a simple pendulum is independent of the mass of the pendulum bob.
Time Period of a Simple Pendulum FAQs
The formula for the time period (T) of a simple pendulum is T = 2π√(L/g), where L represents the length of the pendulum and g is the acceleration due to gravity (approximately 9.8 m/s² on Earth).
The time period of a simple pendulum is directly proportional to the square root of its length. As the length increases, the time period also increases, and vice versa, provided other factors like acceleration due to gravity remain constant.
The time period of a simple pendulum is typically measured in seconds (s) or any other time unit, as long as it is consistent with the units used for length and acceleration due to gravity.
No, the mass of a simple pendulum does not affect its time period. The time period is solely determined by the length of the pendulum and the acceleration due to gravity, while the mass has no influence on it.
No, the amplitude of a simple pendulum, which refers to the maximum angle it swings from its equilibrium position, does not affect its time period as long as the amplitude remains small. The time period remains constant for small amplitude oscillations.
The formula T = 2π√(L/g) for the time period of a simple pendulum is derived under certain assumptions, such as small angle oscillations and negligible air resistance. It provides accurate results for most practical scenarios but may have limitations in extreme conditions or situations deviating from these assumptions.
Yes, the time period of a simple pendulum can be affected by the gravitational field strength. In different locations on Earth or on other celestial bodies, where the acceleration due to gravity varies, the time period of a simple pendulum will also be affected accordingly.
In ideal conditions, the length and acceleration due to gravity are the primary factors that affect the time period of a simple pendulum. However, in real-world scenarios, factors such as air resistance, friction at the pivot point, and structural characteristics of the pendulum may also have a minor influence on the time period.