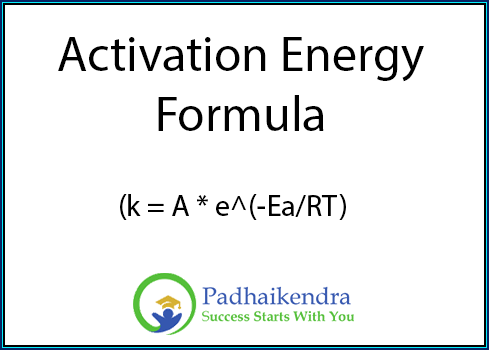
The activation energy of a chemical reaction can be calculated using the Arrhenius equation:
k = A * e^(-Ea/RT)
Where: k = rate constant A = pre-exponential factor or frequency factor Ea = activation energy R = ideal gas constant T = temperature in Kelvin
Taking the natural logarithm of both sides of the equation gives:
ln(k) = ln(A) – (Ea/RT)
This equation has a linear form, with a slope of -Ea/R and a y-intercept of ln(A). Therefore, plotting ln(k) vs. 1/T should give a straight line, from which the activation energy can be determined by the slope of the line.
Solved Example
Question: The rate of a reaction quadruples when the temperature changes from 290 to 330K. Find the energy of activation of the reaction assuming that it does not change with temperature.
Solution:
Given:
T1 = 290K
T2 = 330K
K2 =4K1
From the Arrhenius equation, we obtain
log k2/k1= Ea/2.303R [T2-T1/T1T2]
by substituting all the values we get,
Ea = 1,103276.8/40
=27,581.9 J/mol
Ea = 27.5819 kJ/mol